Java 程序:计算给定矩阵的迹和范数
在本教程中,我们将学习如何找到矩阵的迹和范数。矩阵中的迹定义为对角元素的和,范数定义为矩阵元素平方和的平方根。但是在继续之前,如果您不熟悉数组的概念,那么请务必查看 Java 中的文章数组。
下面是如何找到矩阵轨迹的图示。
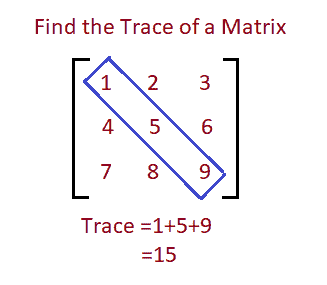
下面是如何求矩阵范数的图示。
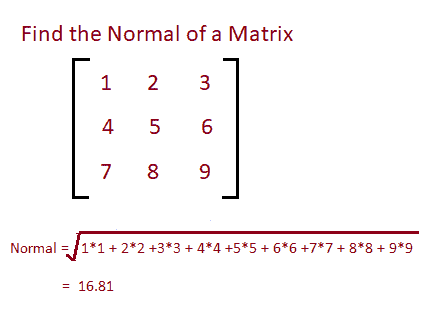
输入:输入矩阵元素:5 4 3 1 2 6 9 8 7
输出:矩阵的轨迹为:14.0
矩阵的范数为:16.88
程序 1:寻找矩阵的迹和范数
在这个程序中,我们将看到当值是用户定义的时,如何找到矩阵的迹和范数。
算法
- 开始
- 为行和列声明变量。
- 要求用户初始化行和列。
- 声明一个矩阵。
- 要求用户初始化矩阵元素。
- 打印原始矩阵。
- 声明两个变量来计算矩阵的迹和范数。
- 将这些变量初始化为零。
- 使用两个 for 循环来计算矩阵的轨迹。
- 使用第一个 for 循环遍历行。
- 使用第二个 for 循环遍历列。
- 使用 if 条件检查行号和列号是否相同。
- 如果相同,则计算每次迭代中的跟踪。
- 打印矩阵的跟踪值。
- 现在,要再次计算矩阵的范数,循环使用两个。
- 使用第一个 for 循环遍历行。
- 使用第二个 for 循环遍历列。
- 计算每个数字的平方,并在每次迭代中更新平方变量。
- 现在,求上面计算的平方的平方根。
- 打印结果。
- 停止
下面的程序演示了如何找到矩阵的迹和范数。
/*JAVA PROGRAM TO FIND THE TRACE AND NORMAL OF A MATRIX*/
import java.util.*;
public class Main
{
public static void main(String []args)
{
///Take input from the user
Scanner sc=new Scanner(System.in);
int m,n; //Matrix Row and Column Declaration
System.out.println("Enter the number of rows: \n");
m=sc.nextInt(); //Matrix Row Initialization
System.out.println("Enter the number of column: \n");
n=sc.nextInt(); //Matrix Column Initialization
int arr[][]=new int[10][10]; //Matrix Size Declaration
System.out.println("Enter the elements of the matrix: ");
for(int i=0;i<m;i++) //Matrix Elements Initialization
{
for(int j=0;j<n;j++)
{
arr[i][j]=sc.nextInt();
}
}
//Print the original Matrix
System.out.println("The elements in the original matrix are: ");
for(int i=0;i<m;i++)
{
for(int j=0;j<n;j++)
{
System.out.print(arr[i][j]+" "); //Print the matrix elements
}
System.out.println("");
}
double sum=0; //Declare and initialize the trace variable
double square=0; //Declare and initialize the normal variable
//Find the trace of the matrix
System.out.println("The Trace of the above matrix is ");
for(int i = 0; i < m; i++)
{
for(int j = 0; j < n; j++)
{
if(i == j)
{
sum = sum + (arr[i][j]); //Calculate the trace in each iteration
}
}
}
System.out.println(sum); //Print the trace of the matrix
//Find the normal of the matrix
System.out.println("The Normal of the above matrix is ");
for(int i = 0; i < m; i++)
{
for(int j = 0; j < n; j++)
{
square = square + (arr[i][j])*(arr[i][j]); //Calculate the normal in each iteration
}
}
double result = Math.sqrt(square);
System.out.println(result); //Print the normal
}
}
输入行数:3 输入列数:3 输入矩阵的元素:1 2 3 4 5 6 7 8 9 原矩阵中的元素为: 1 2 3 4 5 6 7 8 9 上述矩阵的迹为 15.0 上述矩阵的范数为 16.881943016134134
程序 2:寻找矩阵的迹和范数
在这个程序中,我们将看到当值被预定义时,如何找到矩阵的迹和范数。
算法
- 开始
- 声明并初始化矩阵。
- 打印原始矩阵。
- 调用一个方法来计算矩阵的轨迹。
- 在该方法中声明一个变量和,并将其初始化为 0。
- 当对角线值遇到时,增加总和。
- 显示总和。
- 现在,调用一个方法来计算矩阵的范数。
- 声明一个变量 square 并将其初始化为 0。
- 计算每个数字的平方,并在每次迭代中更新平方变量。
- 现在,求上面计算的平方的平方根。
- 打印结果。
- 停止
下面的程序演示了如何找到矩阵的迹和范数。
/*Java Program to find the trace and normal of a matrix*/
import java.io.*;
public class Main
{
//To Find the normal of a matrix
public static void findNormal(int[][] arr)
{
double square = 0, result = 0;
System.out.println("The Normal of the above matrix is ");
for(int i = 0; i < arr.length; i++)
{
for(int j = 0; j < arr[0].length; j++)
{
square = square + (arr[i][j])*(arr[i][j]);
}
}
result = Math.sqrt(square);
System.out.println(result);
}
//To Find the trace of a matrix
public static void findTrace(int[][] arr)
{
double sum = 0;
System.out.println("The Trace of the above matrix is ");
for(int i = 0; i < arr.length; i++)
{
for(int j = 0; j < arr[0].length; j++)
{
if(i == j)
{
sum = sum + (arr[i][j]);
}
}
}
System.out.println(sum);
}
// Driver code
public static void main(String args[]) throws IOException
{
int arr[][]
= { { 2, 9, 8 }, { 7, 6, 4 }, { 3, 9, 2 } }; //Matrix Declaration and Initialization
System.out.println("Original Matrix");
for(int i = 0; i < arr.length; i++)
{
for(int j = 0; j < arr[0].length; j++)
{
System.out.print(arr[i][j]+ " ");
}
System.out.println();
}
System.out.println();
findTrace(arr); //Find the Trace of the Matrix
System.out.println();
findNormal(arr); //Find the Normal of the Matrix
}
}
原始矩阵 2 9 8 7 6 4 3 9 2 T5】上述矩阵的迹为 10.0
上述矩阵的范数为 18 . 36960 . 499999999606